Clarifying Expectations

We’re doing a mini-unit on Probability in my Math Lab class this week. Probability is a tricky thing because it’s hard to predict how much a student has seen in their previous math classes and it’s hard to predict how ‘intuitive’ some students will find it. Today was our first day, so I wanted to take it slow: I wanted to explore how to find the probability of an event by examining the probability space. This breaks down into two separate sub-topics: situations where you already know all possible combinations, and situations where you first have to generate all possible combinations.

I designed the lesson so that we started by performing an experiment – I had every student write down the following on an index card: their name, their age, and their favorite number between 1 and 10. I collected everything, then asked questions around “If I pick a card at random, what’s the probability that the person I pick is…. a boy; a girl; is under 21; wears glasses”. These are all things students can answer from observation. Then I asked “Whats the probability that the card has a favorite number of 5?”, which they can’t answer by observation alone: I have to actually go through the cards and say what everyone wrote down. As I do this, students make a frequency chart – using the chart, we answer the question, then talk about what it means for something to be ‘more probable’ vs ‘least probable’. The whole time, I’m emphasizing the context behind the numerator and denominator of a probability fraction – what we care about / total possibilities (sidenote: I still haven’t found a good catch-all for how to think about the numerator. I shuffle between “What we care about”, “the event happening”, “number of ways to win”, and a few other things depending on the context).

After a bunch of explicit full-group and small-group practice based around this experiment, I give them this problem:

These answers looked familiar – they were fractions just like what we had been doing the last 10-15 minutes as a class. They followed the ‘What we care about/total possibilities’ model. Most worked through this with ease. If there was a mistake, most students forgot to also change the total number of students in problems 14, 15, and 16 (more concretely: before the new student, there are 11 students in the class, so the denominator for #s 1-13 should be 11. After the new student, there are 12 students in the class, so the denominator for #s 14-16 should be 12. Most students left it at 11, even though they correctly changed the numerator)

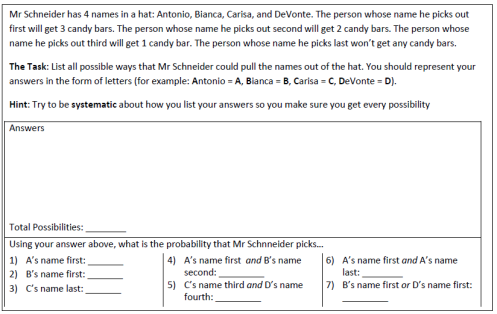
After we checked this problem, I had them flip their paper over, where they saw this problem:

The goal of this problem is to introduce a situation where students must first generate the probability space (ie: find all possible combinations), and only then can they start thinking about probability. I wanted this problem to be the catalyst that forced them to think about systematic ways of listing things. My plan was to model a few possibilities, give them some ambiguous clues as to how to think systematically, let them explore independently and privately acknowledge the students who seemed to have found a system for counting, and then have those students share their strategies with the class. I didn’t tell them how many total possibilities there were, but I did tell them there were more than 16 and less than 30.

Here’s where I had this strange reflective moment that inspired this post

As I found myself describing this second task – listing all possible combinations – I found myself also clarifying my expectations for the purpose of their work. I found myself saying “I’m not expecting you to know the answer right away or to be able to see this immediately. I expect you to start listing possibilities in whatever way you can, but then I hope you find yourself looking for patterns or trying to organize things so that you’re sure you don’t miss any and you’re sure you don’t repeat any”. By the time I was teaching this lesson for the third time today, this speech evolved into something like this: “This task isn’t like the questions on the other side of your sheet where we had practiced and I was expecting you to know the answers right away. This isn’t something we’ve done before, and I’m purposefully letting you explore a little bit before I offer some more guidance. I’m not expecting you to see the answer right away because I want you to give this a try and see if you can find patterns or some clever way to organize your work. But again – the goal is to try something so we can talk about it, not to get the definite right answer right away”

Explicit practice is something we do a lot of in class. Problems for the purpose of exploration and discussion are also something we do a lot of in class. This isn’t the first time I’ve layered both in the same lesson: problems for practice and clarification, then problems for investigation and discussion. BUT – this is the first time I can remember being aware of the difference as class was happening and this is the first time I can remember explicitly describing this difference in expectation to my students. And, as I think about it now, this is also the first time I’ve realized how much of an impact this could have on how my students approach this task. In the past, I’ve probably glided seamlessly from one type of problem to another – “Alright, you guys feel confident about those ones? Let’s try these” without even realizing that my purpose behind the two sets of problems may be fundamentally different.

Being aware of my own expectations and communicating them to students is something I’ve been doing to help with classroom management and class culture, but I think today’s the first time I was aware enough to realize that I also have expectations of how students will react to problems or questions (“They should be able to do this on their own”, “They should do fine up to this step, then we’ll have to regroup”, “We’ll use these to frame our class discussion”), that those expectations can change rapidly mid-lesson, and that these expectations are important for the mindset my students have as they approach these problems. I wonder how many lessons I’ve given where I’ve had this transition and never mentioned the shift in expectation to my students. I wonder if it made a difference in the past – if students struggled with these investigative questions purely because I didn’t make clear that I was no longer expecting an immediate answer. If they shut down quicker because they weren’t sure how to solve it – or, even worse, if I was more impatient because I transitioned from practice questions to investigation questions without even realizing it myself.

Anyway – in this particular lesson, students took risks and started to write down possibilities even if they weren’t sure that they were on the right track; they were receptive when I had a fellow student explain one of their methods for counting; and they participated in the ‘how…? why…?’ discussion that happened afterwards. I wonder if the same thing would have happened if I had just given them the task without clarifying that this was a different type of problem with a different expected outcome. I wonder if students would have begun the task thinking it was just more practice and more familiar, only to become frustrated and shut down more easily. I wonder how many times I’ve accidentally done this in the past too.